Discover in depth the 10 meta-cognitive principles higher math folks use to absorb math quickly, overcome intellectual plateaus while having a great time.

Most guides on mathematical learning are either too shallow, too narrow or too K-12, so we decided to create a better one instead.

Yes! This is exactly what I've been looking for!

So here's what happened...

As a tutoring agency covering the juncture between lower and higher mathematics, we often cater to a wide range of students with various levels and backgrounds in mathematics.

But because of that, there's also a troubling trend which we can't help but notice...

Namely,

We observed that the vast majority of students really aren't well prepared to tackle mathematics beyond the K-12 level at all!

For example, they would:

Throw their hands up when theoretical understanding is required
Have a hard time with grasping abstract, higher-level concepts despite numerous trials
Experience difficulties carrying out logical deductions from one end to another
Struggle with problems where they have to come up with methods or theory on their own

But then, that's when we had a déjà vu,

because there was a time where we were just like them as well:

For example, we would:

Look at a proof or a mathematical passage, and have no clue what we just read
Look at a mathematical problem, and struggle for hours without even a systemic plan to go about it
Try to force learn a higher math topic — only to forget most of it in the next few days
Get angry at ourselves and ask "why do we need this?" — when no result is forthcoming

So, what is it that we and many others were missing?

"Higher Mathematical Learning Skills"

But you may ask, "how could that be!?" After all, most of us have at least 10 years of math under our belt by the time we finish high school.

But here's the thing...

"Our system — here in North America at least — is primarily set up to teach the most miniaturized form of mathematics, aiming for almost the lowest common denominator."

Mathematics with neither the whys nor the rigor
Mathematics without much higher-level abstract thinking or deduction
Mathematics as tools and algorithms
Mathematics done for you in a box — with little room for struggle, exploration and invention

So no wonder why our society has become so mathematically fragile — with most of us having little clue as how to tackle mathematics except the simplest.

Or to put it more bluntly, we're living in the "mathematical matrix", without realizing that we're being mathematically domesticated as we speak.

And because of that, our team decided to embark on a journey — to be on the lookout for a guide that would solve that problem.

We also started to reminisce extensively on our own experience, and to do some research around the topic pretty much right away.

However. as we delve deeper into the researching process,

We also realized that there is actually not a lot of resources when it comes to higher mathematical learning!

Instead, what we did find are stuffs such as:

1-page articles on general tips and tricks for studying K-12 math
Popular guides on introduction to proof and problem solving
Research papers and theses on the state of mathematical education
Essays by educators reflecting on various teaching methodologies

Essentially, we weren't able to find a guide on math learning that is:

Well-structured
Well-researched
Higher-math-focused
Succinct
Scalable beyond basic math

Principle-based
Practical
Insightful
Comprehensive
Actionable

So, we decided to do the obvious thing...

— which is to write the guide ourselves.

And then, after 5 months of:

Researching
Brainstorming
Redrafting
Designing
Other technical undertaking...

We finally managed to compile our research and experience into a guide similar to the one described above...

$Higher Math Guide P51$

— where we reveal 10 powerful, rarely-elaborated principles on higher mathematical learning.

Introducing...

The Definitive Guide to Learning Higher Mathematics

— 10 Principles to Mathematical Transcendence

A 10-chapter, full-length 85+ page eBook — with each chapter focusing on a fundamental principle of mathematical learning at a higher level.
A dense, primarily non-technical guide in higher mathematical learning — where structured learning principles meet actionable tips to saving years of wasted and misguided effort.

$Higher Math Guide Cover iPad$

So just what kind of principles and concepts are we talking about?

Well, here's the table of contents for the record:

$Higher Math Learning Guide ToC1$

$Higher Math Learning Guide ToC2$

$Higher Math Learning Guide P49$

For example, for each chapter:

A learning principle is first introduced by exploring the research and the rationale behind it.
Their applicability to higher math learning is then elaborated — through a series of actionable tips, tricks and strategies.
From time to time, some ancillary information is supplied along the way to fill in the knowledge gaps.
And when all is done, the chapter is then concluded with a summary of all the key takeaways.

And while the guide is primarily structured to be non-technical, it also strikes a balance to include a wide range of examples from fields such as:

Calculus
Real analysis
Complex analysis
Linear algebra
Number theory

(to be sure, these are examples that could very well be covered either before or during the first year of a typical math undergraduate study.)

$Higher Math Learning Guide P25$

In fact, most of the ideas and concepts featured in the guide are research-backed,

yet framed in an entirely original way.

These include:

Powerful pedagogical concepts not made explicit elsewhere
Not-so-obvious, controversial "cognitive buzzwords"
Cognitive concepts and techniques coined at this side of the corner

$Higher Math Guide P27$

If anything, there's a chance that you might find some of the advice there counter-intuitive or shocking.

Heck, it might even change your conception of the role of a mathematical learner/educator in our society!

$Higher Math Guide P36$

Along the way, we've also introduced a series of modules to make the guide more complete and insightful:

Definition modules
Pro-tip modules
Did-you-know modules
Famous mathematicians and quotes

And some other notable features such as:

Extensive annotations
Illustrative diagrams
Linkable table of contents
9-page linkable index
Other features making the presentation more intuitive and more insightful

$Higher Math Guide P44$

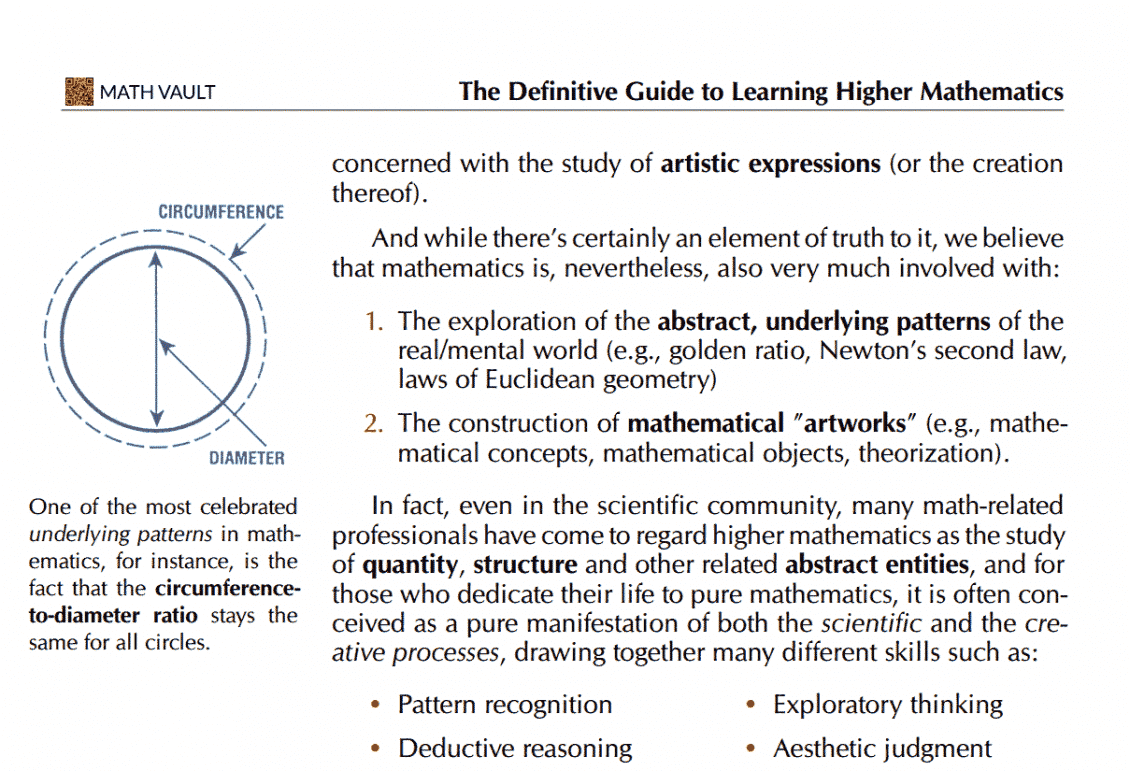
And of course. The overview wouldn't be complete without some memorable lines from the guide such as:

"Taking responsibility in choosing our own math materials is better than getting struck with resources which we don't find particularly useful."

In other words, if you're interested in:

Absorbing abstract concepts faster with better retention
Solving problems more efficiently while having fun at it
Becoming more mathematically mature and independent
Making higher mathematics an integral part of your well-being

then The Definitive Guide to Learning Higher Mathematics would be a good place to start your awakening!

$Higher Math Guide P67$

$Higher Math Learning Guide$

In fact, this is the guide we wished we've had — before even starting our journey in higher mathematics!

Alright. Time for some questions.

What are the pre-requisites for this guide — if any?

How self-sufficient / self-contained is The Definitive Guide to Learning Higher Mathematics?

How come this project took 5 months to complete?

How is this guide different from other math learning guides on the market?

Will this guide teach me to become a better problem solver?

Will this guide make me a better mathematical thinker?

Will this guide teach me any new mathematics?

Will this guide train me to become a better mathematician — amateur or professional-wise?

Get the most out of mathematics with The Definitive Guide to Learning Higher Mathematics today.

Yes! I'd like to grab my copy and optimize my higher math learning potential today.

P.S. — Congratulations! Looks like you've just made it through the end of our presentation! For any question, just drop us a line at [email protected] and we would be more than happy to help you out!